ere, we show you how this lemma lets us derive the basic Black-Scholes partial differential equation. For convenience, we restate Ito’s lemma applied to the call option:

Ito’s Lemma: If dS = mSdt + sSdz, and C(S,t) is the price of the call option, then

You should note that the drift rate of the call is (1/C) times the term multiplying dt:

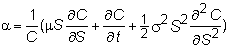
and the volatility is (1/C) times the term multiplying dz:

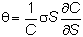
Stock Options

Let S be the stock and C the call option on the stock.  The lemma says that the excess drift rate divided by the volatility should be equal for the stock and the call option.  This means that for a non-dividend paying stock:

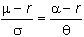
All you have to do is substitute for a and q and simplify to construct the Black-Scholes PDE (see the next topic Construction of the Black-Scholes).  This is:

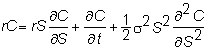
Finally, the required adjustment for options defined on an asset that pays a continuous constant dividend requires that the equation be defined as: