2.2
Option Valuation: A Riskless Hedge Example
|
|
n
this topic, we show that you can use the one-period binomial model to value an
option by creating a riskless portfolio using the stock and an option.
Here, riskless means that the portfolio has a known value at the end of
the period, no matter what happens to the stock price.
If
the portfolio is riskless, then we know its current value; it is simply the
future value discounted by the risk-free interest rate.
Then, since we know the portfolio value and the stock price, we can
determine the option price.
Why
are we doing this? The answer comes
from the general principle of valuation,
which says that the value of an asset is the present discounted value of all
future cash flows from the asset. To
value an option, we then need to determine two things: the future cash flows and
the discount rate.
For
a European option, the future cash flows are easy; for example, for a call
option, the cash flow is 0 if the future stock price is less than the strike
price, and equals the future stock price minus the strike price otherwise.
What
about the discount rate? Here lies
the problem. We cannot use the
risk-free interest rate, since the future cash flows are not risk-free; they
depend on the unknown future stock price. If people are risk-averse, then they
will hold risky securities only if they can get a return greater than the
risk-free interest rate. In fact,
much of the problem in valuing risky securities is determining the appropriate
discount rate, as in the capital asset pricing model. The fundamental riskless
hedge argument solves the problem of determining the discount rate, since we
know how to discount the riskless portfolio.
An
example shows you how to create a riskless portfolio.
You can work through the example in this topic both numerically and
graphically by using the Binomial Delta
Hedging subject in Option Tutor.
Let S denote the current stock price and assume that at the end of one
period the stock value is either 10 or 40.
We will study European put and call options with a strike price,
X, of 20 when the risk-free interest rate is zero.
The
future values of the stock and the options are depicted in the "tree"
in Figure 2.1,
Figure 2.1
One-Period Binomial Tree
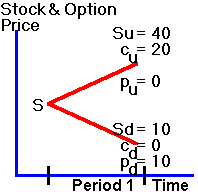
where,
given an uptick, u, Su
is the realized stock price, Cu is the realized call option value, and Pu
is the realized put option value.
You know, for instance, that Pu
is zero
since the put option is worthless when S =
40 > X = 20.
Now
suppose you form a portfolio:
Buy
2 stocks;
Sell
3 call options
Consider
what happens at the end of the period if a downtick occurs.
Since the stock is worth 10, the call option finishes out-of-the-money
and the trader you sold the call option to would not exercise it. The final payoff from your portfolio is 20, which is the
value of the two stocks.
Suppose
an uptick occurs. Each stock is
worth 40, but the calls would now be exercised against you.
You would be required to give three stocks to the person who bought the
options, and you would receive 20 for each stock.
Therefore, your final position would be:
+
80 from the stock you hold;
+
60 from the three options being exercised;
-
120 to buy the three stocks to give to the option exerciser;
which
leaves you with 20.
Therefore,
no matter what the state (or final value of
the stock), you will end up
with 20 at the end of the period as indicated in Figure 2.2.
Figure 2.2
A Riskless Portfolio
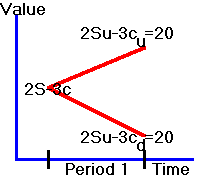
You
now have a riskless portfolio. The
present value of this portfolio is 20 since we have assumed that the risk-free
interest rate is zero.
This
means that the portfolio that is long 2 stocks and short 3 call options must
trade for a price equal to 20. If
not, there is an arbitrage opportunity. To
see this, suppose that you could sell such a portfolio for more than 20. That is, 2S - 3C
> 20, where S is the stock price and C
is the call price. Then, you can
profit from selling this portfolio. You
receive more than 20 from the sale, but lose at most 20 at the end of the
period, which ensures you of a profit. Similarly,
if you could buy this portfolio for less than 20, 2S
- 3C < 20, then you can profit
from buying it.
Each
of these situations presents an arbitrage opportunity (i.e., the ability to make
a sure return for zero wealth). The
only price at which arbitrage is not possible is if you can buy or sell this
portfolio at 20, (2S - 3C
= 20).
Since
2S - 3C = 20, we know that C
= (2S - 20)/3, so if you know the price of the underlying stock, you
know the value of the call option.
Puts
can be priced in the same manner (by considering portfolios in which you buy
stocks and buy puts). You may want
to verify that a portfolio consisting of one stock and three puts is worth 40 at
expiration. Since the
portfolio costs S + 3P, we get S + 3P
= 40, so P = (40 - S)/3 is the put
price.
Positive
Interest Rate
Our
analysis so far assumes that the risk-free interest rate is zero.
Suppose instead the interest rate is some positive amount. This assumption changes the analysis a little.
No longer is 20 an arbitrage-free price, because now there exists a
better opportunity.
To
see why, suppose at the beginning of the period you could sell 2S
- 3C at 20. You can now profit from selling this portfolio and investing
the proceeds at the risk-free interest rate.
Let r = 1 + the risk-free
interest rate. At the end of the
period you will receive 20r >20,
and pay out 20.
To
eliminate this arbitrage opportunity, it must be the case that in the presence
of a positive risk-free rate of interest 2S
- 3C = 20/r. Therefore the
arbitrage-free option prices are obtained as before with this adjustment, C
= (2S - 20/r)/3 and P
= (40/r - S)/3.
The
Binomial Delta Hedging subject in Option Tutor lets you rework this
example for different interest rates.
Summary
We
have shown how to value an option by constructing a riskless portfolio. We then use the fact that we know how to discount a riskless
portfolio to obtain the value of the option.
A
second approach to valuing options is to form a synthetic
option exampleSOE_BIN from the
underlying asset and a bond. An
example of this approach is presented in the next topic.
 office (412)
9679367
office (412)
9679367