urrency options are important instruments for managing exchange rate risk.  As a currency trader, you are interested in knowing:

1.  how your position is exposed to changes in exchange rates, changes in interest rates for both the strike and the deliverable currency, and changes in exchange rate volatility;

2.   how you can hedge your position against these sources of risk.

The hedge parameters provide the answers to 1., and using these parameters traders can identify the appropriate option trading strategy to answer 2.  Online, you can graph the hedge parameters as a function of any of the underlying variables.  The mathematics underlying these graphs is summarized here; it is the same as for the continuous dividend yield model, except that rd replaces the dividend yield.

The call option pricing formula is

and since

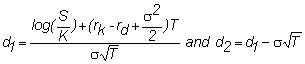
The variables that affect the option price are

S = exchange rate

K = the strike price

s = the volatility of S

T = the time to maturity

 = the risk-free interest rate in the strike country

r_d = the risk-free interest rate in the deliverable country

By calculating the derivative of the call price with respect to each variable, we obtain the hedge parameters.  You may want to compare these to Comparative Statics, in Chapter 9, topic 9.3.

Delta

For a call option and a put option, respectively, delta is given by:

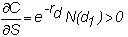
and

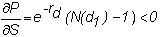
The delta of the call tells you how the value of the call changes if there is a small change in the exchange rate S. 

For example, if you have written 1,000 currency call options that have a delta of 0.85, then you need to be long 850 units (= 0.85*1,000) of the bond in the deliverable currency to create a delta-neutral position.  If delta changes to 0.86, to remain delta-neutral,  you would have to buy an additional 10 units (= (0.86-0.85)*1,000) of the bond.

You should observe that because maintaining the hedge requires that you hold the zero-coupon bond in the deliverable currency, you are earning a continuously compounded riskless rate of interest equal to rd.  The mathematical details associated with forming a riskless hedged portfolio are provided in Chapter 12, topic 12.4, Application:  Currency Options.

Gamma

The gamma of a currency  option measures how the delta changes with S.  This provides useful insight into the potential frequency of rebalancing that is required to maintain some target delta.  That is, the gamma tells you how far you are from some target hedge. For either a call or a put option, gamma is

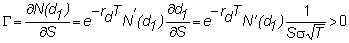
Online, you can see that a position is most sensitive to changes in delta when it is at-the-money.

Rho

Rho measures the change in the call option with respect to a change in the riskless interest rate.  For currency options, this is sometimes called the interest rate delta.  There are two rhos, one with respect to the strike country's interest rate, and the other with respect to the deliverable country's interest rate.

For the strike currency:

and for the deliverable currency's risk-free interest rate:

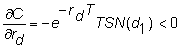
Vega (Lambda or Kappa)

The vega measures the effect of a change in the volatility of the exchange rate.  It is given by

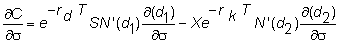
and thus,

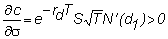
In Chapter 11 you will see the Black-Scholes option pricing model extended to value options on futures in topic 11.3, Pricing of Options on Futures.